The Golden Mean and Fibonacci Series

Some 20th-century composers and writers have been interested in the "golden mean" or "golden section," a proportion used for centuries in art and architecture to obtain aesthetically pleasing designs.  The golden mean is the division of a whole into two unequal parts such that the ratio of the smaller to the larger is the same as that of the larger to the whole.

To understand this ratio, consider a line AC with line segments AB and BC.  If the proportion of AB to BC is the same as the proportion of BC to the whole line, AC, then AC is segmented according to the golden mean.  This relationship can be expressed as:

Integers (whole numbers) that approximate the golden mean can be generated by means of a Fibonacci Series, an endless series of numbers in which each number is the sum of the previous two.  The farther you go in the sequence, the closer you get to the true value of the golden mean. The most obvious way that this ratio can be applied musically is in the proportions of a musical form.  For example, the beginning of "Minor Seconds, Major Sevenths," from Bartok's Mikrokosmos, could be subdivided in this way:


There is some evidence that Bartok (and Debussy) used the golden mean not only in formal proportions but in other aspects of his music as well, and this is also true, if to a lesser extent, of some other 20th-century composers.

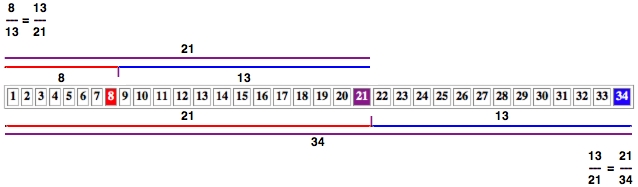
Musical frequencies are based on Fibonacci ratios

Notes in the scale of western music have a foundation in the Fibonacci series, as the frequencies of musical notes have relationships based on Fibonacci numbers:

Fibonacci
Ratio		 Calculated
Frequency		 Tempered
Frequency 		Note in
Scale		 Musical
Relationship		 When
A=432 *		 Octave
below		 Octave
above
1/1 440 440.00 A Root 432 216 864
2/1 880 880.00 A Octave 864 432 1728
2/3 293.33 293.66 D Fourth 288 144 576
2/5 176 174.62 F Aug Fifth 172.8 86.4 345.6
3/2 660 659.26 E Fifth 648 324 1296
3/5 264 261.63 C Minor Third 259.2 129.6 518.4
3/8 165 164.82 E Fifth 162 (Φ) 81 324
5/2 1,100.00 1,108.72 C# Third 1080 540 2160
5/3 733.33 740.00 F# Sixth 720 360 1440
5/8 275 277.18 C# Third 270 135 540
8/3 1,173.33 1,174.64 D Fourth 1152 576 2304
8/5 704 698.46 F Aug. Fifth 691.2 345.6 1382.4

The calculated frequency above starts with A440 and applies the Fibonacci relationships.  In practice, pianos are tuned to a "tempered" frequency to provide improved tonality when playing in various keys.

* A440 is an arbitrary standard.  The American Federation of Musicians accepted the A440 as standard pitch in 1917.  It was then accepted by the U.S. government its standard in 1920 and it was not until 1939 that this pitch was accepted internationally.  Before recent times a variety of tunings were used.  It has been suggested by James Furia and others that A432 be the standard.  A432 was often used by classical composers and results in a tuning of the whole number frequencies that are connected to numbers used in the construction of a variety of ancient works and sacred sites, such as the Great Pyramid of Egypt.  The controversy over tuning still rages, with proponents of A432 or C256 as being more natural tunings than the current standard.